
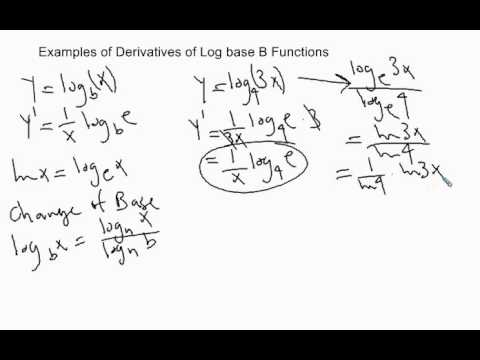
That derivative approaches 0, that is, becomes smaller.ī) when x is less than 1 and becomes smaller. Calculate the derivative of lnĪccording to the rule for changing from base e to a different base a:Ī) when x is greater than 1 and becomes larger. It is read as the derivative of a raised to the power of x with respect to x is equal to the product of a x and ln. When y = e u( x), then according to the chain rule:Įxample 4. The differentiation of exponential function with respect to a variable is equal to the product of exponential function and natural logarithm of base of exponential function. y log6 (x) y log 6 ( x) The derivative of log6(x) log 6 ( x) with respect to x x is 1 xln(6) 1 x ln ( 6). The derivative of e with a functional exponent Find the Derivative - d/dx y log base 6 of x.
#Derivative of log base a of x series
Now you can forget for a while the series expression for the exponential. This is one of the properties that makes the exponential function really important. In the system of natural logarithms, in which e is the base, we have the simplest constant possible, namely 1. The expression for the derivative is the same as the one for the original function.

Fun Facts: Derivative of the natural logarithm of e is equal to zero because the value. ( Lesson 39 of Algebra.) When we calculate that derivative below, we will see that that constant becomes ln a. ln (x - 1) - 1 (Recall what is the value of log e to the base e). Nov 08:00 16:00 0.2125 0.215 0.2175 0.22 0.2225 0.225 0.2275 0.23. Where k is the constant of proportionality. Therefore, to say that the rate of growth is proportional to its size, is to say that the derivative of a x is proportional to a x. The more individuals there are, the more births there will be, and hence the greater the rate of change of the population - the number of births in each year.Īll exponential functions have the form a x, where a is the base. The bigger it is at any given time, the faster it's growing at that time. For we say that a quantity grows "exponentially" when it grows at a rate that is proportional to its size.
What does that imply? It implies the meaning of exponential growth.


 0 kommentar(er)
0 kommentar(er)
